Mathic
Between all languages and cultures there exists a universal truth in math.
Within math, there exists fundamental constants of nature and sets of numbers that seem to define the world around us. These universal mathematic truths are a humbling reminder of the illusory nature of the cultural disparities that we have fabricated.
Math, as a universal fundament, could be viewed as the potential for a greater truth that transcends human differences. The goal of Mathic is to embody these mathematic truths in architectural forms, creating a peaceful space to ponder the nature of our own existence.
By using fundamental constants and sets to define form, an homage to our universal truths takes shape. Each of the structures in Mathic are defined by a certain mathematical property, function, or set.
Mathic was commissioned for design by a private client
The supports of the bridge to the main approach are golden spirals - directly linked to the Golden Angle in that their spiral growth factor is defined by the Golden Ratio.
The fountain on the main approach is a rotation of the Mandelbrot Set. By only iterating a few times over the equation that defines the unique set, a smooth approximation of the set is formed in 2D, then revolved to form a 3D shape that defines the fountain’s form.
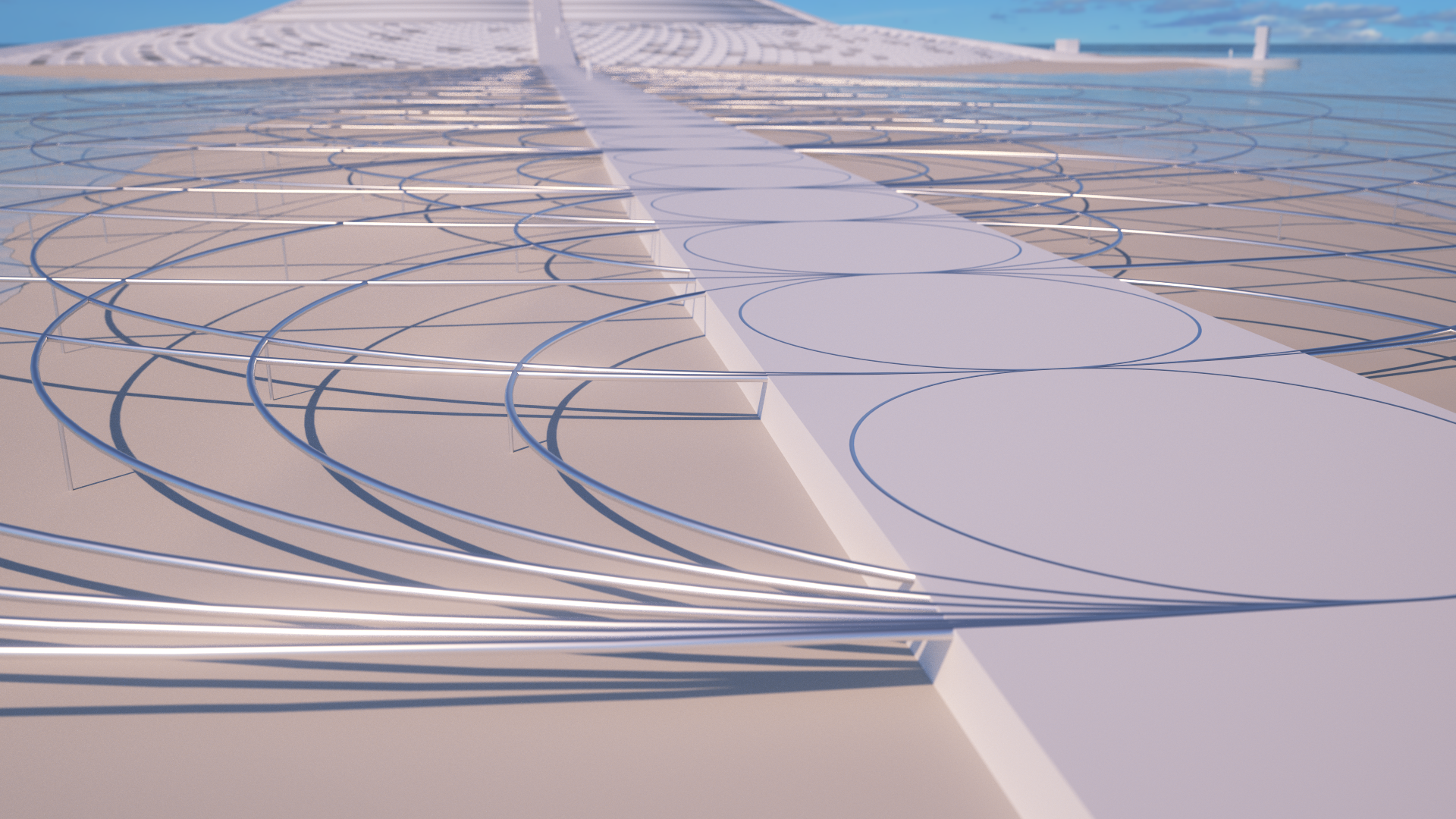
These rings along the main approach form the shape of a prime sieve. Each ring represents a certain component of a multiple of a number. The smallest rings are one unit in diameter, and represent multiples of one. Points where many rings meet represent a number with many divisors, while points with only one intersection could represent prime numbers.
The upper steps to the main structure are formed from offsets of the distance field of the main structure. Each step is an offset of the distance field equation that defines the main structure (explained in more detail below). The coloring of a step is grey if its descending step count is prime.
The main structure is defined by a Kaleidoscopic Iterative Function, which is a type of fractal that uses a series of reflections and rotations to create a distance field.
For a visual explanation, this video illustrates the process: https://vimeo.com/604486462/b3e9368daf
The Mandelbrot set forms this satellite structure. By measuring the maximum bounding box of each iterated point and extruding that point in z-space, it forms a third-dimension visualization based on the Mandelbrot set’s iterations.
© Future Wife, LLC